JEE 2018 :: Mathematics (2018) :: Solved questions (2018)

• Mathematics (2018) - Solved questions (2018)

Let X  be the set consisting of the first 2018 terms of the arithmetic progression 1,6,11,..... and Y be the set consisting of the first 2018 terms of the arithmetic progression 9,16,23,.... Then , the number of elements in the set X υ Y is.....

Answer:

Explanation:

Here X= {1,6,11,.......,10086}

                      [  $\because a_{n}=a+(n-1)d$ ]

  and Y= {9,16,23,...........,14128}

           X ∩ Y={16,51,86......}

$\because$ t_n of X∩ Y is less than or equal to 10086

     t_n = 16+(n-1)35 ≤ 10086

$\Rightarrow$    n ≤ 288.7

                    n=288

  $\because$    n(X ∩ Y) =n(X) +n(Y) - n(X ∩ Y )

                      n (X ∩ Y) = 2018+2018-288

                                     =3748

The number of 5 digit numbers which are divisible by 4, with digits from the set {1,2,3,4,5} and the repetition of digits is allowed, is...........

Answer:

Explanation:

A number is divisible by 4 if last 2 digit number is divisible by 4

                 Last two digit number divisible by 4 from {1,2,3,4,5} are 

                     12,24,32,44,52

  The number of 5 digits number which are divisble  by 4 , from the digit (1,2,3,4,5)  and digit is repeated is

            5× 5× 5× (5× 1) =625

The value of    $((\log_{2}{9})^{2})^{\frac{1}{\log_{2}{}(\log_{2}{9})}\times\frac{1}{(\sqrt{7})^{\log_{4}{7}}}}$  is............

Answer:

Explanation:

$((\log_{2}{9})^{2})^{\frac{1}{\log_{2}{}(\log_{2}{9})}\times\frac{1}{(\sqrt{7})^{\log_{4}{7}}}}$

   =   $(\log_{2}{9}^{})^{\frac{2\log_{}{2}}{\log_{2}{9}}\times}7^{\frac{1}{2}\log_{7}{4}}$

   =   $(\log_{2}{9})^{\log_{\log_{}{2}}{9}_{}^{2^{2}}}\times 7^{\log_{7}{2}}$

     = 2²× 2=8

Let P₁: 2x+y-z=3    and P₂ : x+2y+z=2 be two planes. Then, which of the following statements (s) is (are) TRUE ?₁

Answer:

Explanation:

We have

    P₁: 2x+y-z=3        and

    P₂ : x+2y+z=2

  Here,   $\overrightarrow{n_{1}}=\hat{2i}+\hat{j}-\hat{k}$

   and    $\overrightarrow{n_{2}}=\hat{i}+\hat{2j}+\hat{k}$

 (a)  Direction ratio of the line of intersection of P₁ and P₂  is θ  $\overrightarrow{n_{1}}\times\overrightarrow{n_{2}}$

i.e

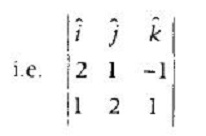
   = $(1+2)\hat{i}-(2+1)\hat{j}+(4-1)\hat{k}$

   =  $3(\hat{i}-\hat{j}+\hat{k})$

    Hence, statement a is false

(b) We have

         $\frac{3x-4}{9}=\frac{1-3y}{9}=\frac{z}{3}$

$\Rightarrow$   $\frac{x-\frac{4}{3}}{3}=\frac{(y-\frac{1}{3})}{-3}=\frac{z}{3}$

   This line is parallel to the line of intersection of P₁ and P₂ .

Hence statement (b) is false.

(c)  Let acute angle between P₁ and P₂ be θ

      We know that ,

         $\cos\theta = \frac{\overrightarrow{n_{1} }\overrightarrow{n_{2}}}{\mid\overrightarrow{n_{1}}\mid \mid\overrightarrow{n_{2}}\mid }$

  = $\frac{(2\hat{i}+\hat{j}-\hat{k}).(\hat{i}+2\hat{j}+\hat{k})}{\mid 2\hat{i}+\hat{j}-\hat{k}\mid \mid\hat{i}+2\hat{j}+\hat{k}\mid}$

  =  $\frac{2+2-1}{\sqrt{6}\times\sqrt{6}}= \frac{1}{2}$

    θ =60°

Hence the statement (c) is true

   (d) Equation of plane passing through the point (4,2,-2) and perpendicular to the line of intersection of P₁ and P₂ 

                   3(x-4)-3(y-2) +3(z+2)=0

 $\Rightarrow$    3x-3y+3z-12+6+6=0

   $\Rightarrow$    x-y+z=0

   Now distance of the point (2,1,1)

   the plane x-y+z=0 is

 $D =\mid\frac{2-1+1}{\sqrt{1+1+1}}\mid =\frac{2}{\sqrt{3}}$

   Hence statement (d) is true                      

In a Δ PQR , Let  $\angle PQR=30^{0}$ and the side PQ and QR have lengths 10√3 and 10, respectively . Then , which of the following statement (s) is (are) TRUE?

Answer:

Explanation:

 In  Δ PQR 

          $\angle PQR=30^{0}$

                  PQ = $10\sqrt{3}$    , QR=10

By cosine rule

   $\cos 30^{0}=\frac{PQ^{2}+QR^{2}-PR^{2}}{2PQ.QR}$

  $\Rightarrow\frac{\sqrt{3}}{2}=\frac{300^{}+100^{}-PR^{2}}{200\sqrt{3}}$

  $\Rightarrow$     300=300+100-PR²

  $\Rightarrow$     PR=10

   Since , PR=QR= 10

  $\therefore$     $\angle QPR=30^{0}$   and    $\angle QRP=120^{0}$

Area of ΔPQR = $\frac{1}{2}$ PQ.QR $\sin 30^{0}$

              =  $\frac{1}{2}\times10\sqrt{3}\times 10\times\frac{1}{2}=25\sqrt{3}$

Radius of incircle of

            Δ PQR= $\frac{Area of \triangle PQR }{Semi-perimetre of \triangle PQR}$

 i.e   $r=\frac{\triangle }{s}= \frac{25\sqrt{3}}{\frac{10\sqrt{3}+10+10}{2}}=\frac{25\sqrt{3}}{5(\sqrt{3}+2)}$

  $\Rightarrow$       r= $5\sqrt{3}(2-\sqrt{3})=10\sqrt{3}-15$

       and radius of the circumcircle 

                       $(R)=\frac{abc}{4\triangle}= \frac{10\sqrt{3}\times10\times10}{4\times25\sqrt{3}}=10$

$\therefore$   Area of circumcircle of Δ PQR=  $\pi R^{2}=100\pi$

         Hence, option (b), (c) and (d) are correct answer.

• Mathematics (2018) - Solved questions (2018)